Differential Equations Online Course for Academic Credit
Differential Equations can be best described as "Higher-Level Integration Theory". The simplest Differential Equations have solutions that are simple Integrals as you learned in Calculus II. But very quickly the Differential Equations become more complicated, and so, too, are the solutions. Physicists think of Differential Equations as the equations that get spit out from their analysis of the various physics situations, and thus need to be solved to understand the physics. Unfortunately, most Differential Equations cannot be solved algebraically, but the main focus of classroom/textbook courses is usually to just try to exhaust all of the Differential Equations that can be solved by hand.
| Course Title: | Computational Differential Equations |
| Catalog Number: | DMAT 321 |
| Credits: | 3 Semester Credit Hours |
| Syllabus PDF: | PDF Syllabus for Computational Differential Equations |
| Delivery: | Fully Online, Asynchronous, Self-Paced |
| Click Here to Enroll in DMAT 321 - Computational Differential Equations | |
Our Differential Equations online course via Distance Calculus @ Roger Williams University takes a different approach: what do these differential equations mean? What do their solutions mean? What do their graphical or numerical solutions mean? Using a power tool like Mathematica, we are not bound by just those differential equations that have hand-calculated solutions, but rather all differential equations are fair game, and we investigate the concepts of Differential Equations from a laboratory-science point of view.
The first course in a study of Differential Equations is often called Ordinary Differential Equations; other names for this course include:
- Introductory Differential Equations
- Ordinary Differential Equations
- A First Course in Differential Equations
- Single-Variable Differential Equations
Completion of DMAT 321 - Computational Differential Equations earns 3 academic credit semester hours with an official academic transcript from Roger Williams University, in Providence, Rhode Island, USA, which is regionally accredited by the New England Commission of Higher Education (NECHE), facilitating transfer of credits nationwide to other colleges and universities.
Differential Equations Online Course Introductory Videos
Differential Equations Online Course Introduction
Differential Equations can be thought of as "the task of integration, with (more and more) complications".
Simple algebraic integration of a function f(x) can be re-interpreted in terms of this integral being the solution of a differential equation y' = f(x), and our task is to solve for y - as integration is the "inverse" operation of differentiation, we see that y is the algebraic integral of f(x).
The equation y' = f(x) is the most basic differential equation possible. Quickly we are lead to investigate more complicated forms of equations involving differentiation, for example: y' = y + f(x), which asks: find a function y = y(x) which has the property that its derivative y' is equal to itself y added to a function f(x). Not an easy question before starting the Differential Equations course, but upon completion of this course, such questions - and exponentially more difficult and complex such equations - are answered with skill and understanding.
More than just an "algebraic game involving integrals", the topic of Differential Equations studies not only the algebraic solutions of such equations (when possible!), but also the qualitative understanding of the properties and solutions of these equations.
Traditional Differential Equations courses often are dedicated to learning these "expanded integration techniques" to study the solutions of these equations purely from an algebraic point of view. While this approach has its merits, the types of differential equations encountered "in the real world" (i.e physics, chemistry, engineering, etc) require solution and analysis techniques beyond what is possible via algebra alone.
The course curriculum for our Differential Equations online course is based upon Differential Equations&Mathematica by Carpenter/Davis/Uhl, and utilizes the high-powered computer algebra and graphing system Mathematica™ by Wolfram Research. Engaging the algebraic investigations of the classes of differential equations studied on briefly, the curriculum moves quickly to investigating the much richer concepts accessible via Mathematica™ and its numerical and graphical differential equation solvers, opening up the introductory study of differential equations beyond the traditional textbook on the subject.
Roger Williams University Course Catalog Listing: DMAT 321 - Computational Differential Equations
Course: DMAT 321
Course Title: Computational Differential Equations
Transcript Course Title (30 Characters Max:): Comp Differential Equations
Course Description: A first course in the study of differential equations with emphasis on modern software computational techniques with geometrical and qualitative interpretations. Topics include first, second, and higher-order ordinary differential equations, analysis of forcing functions, Laplace Transforms, convolution integral techniques, Fast Fourier Transforms and data approximations, systems of differential equations, classical algebraic solution methods, power series solutions. [3 Semester Credits]
Prerequisite: Successful completion (C- or higher) of Calculus II or equivalent, or consent of instructor.
E-Textbook: Differential Equations & Mathematica by Davis/Porta/Uhl
Software: Mathematica
PDF Course Syllabus: Detailed Course Syllabus in PDF for DMAT 321 - Computational Differential Equations
DMAT 321 - Computational Differential Equations - Learning Outcomes
- 1. To understand the core construction of the differential equation, and its classification parts
- 2. To understand the role of the forcing function in differential equations
- 3. To understand, observe, and compute the steady state solutions for a differential equation
- 4. To understand, observe, and compute solutions of differential equations with a variety of forcing functions, including DiracDelta, step, oscillatory, and others
- 5. To understand, observe, and compute solutions of differential equations with a variety of forcing functions, including DiracDelta impulses, step, oscillatory, et al.
- 6. To understand and compute solutions of second order differential equations oscillators and how forcing functions affect their solutions
- 7. To understand and compute manual solutions of first and second order differential equations using classical techiques
- 8. To understand and compute with the Laplace Transform method
- 9. To understand and compute graphical and numerical solution methods of differential equations
- 10. To understand and compute solutions of linear systems of differential equations
- 11. To understand and compute polynomial approximations to solutions of differential equations
DMAT 321 - Computational Differential Equations - Syllabus of Topics
1. Getting Started 1.1. Email and Chat 1.2. Learning About the Course 1.3. Required Hardware 1.4. Software Fundamentals 2. Exponential Differential Equations 2.1. Unforced DEs 2.2. Forced DEs 2.3. Steady State 2.4. Personal Finance 2.5. Step Function and Dirac Delta Function 2.6. Tangent Vectors 2.7. Initial Conditions 2.8. Integration Factors 3. Second-Order Differential Equations 3.1. Overdamped and Underdamped Oscillators 3.2. Linear Forced and Unforced Oscillators 3.3. Homogeneous and Inhomogeneous Equations 3.4. Convolution Method 3.5. Characteristic Equations 3.6. Euler's Formula 3.7 Impulse Forcing 3.8 Dirac Delta Convolutions 3.9 Springs and Electrical Charges 3.10 Higher Order Equations 4. Laplace Transforms 4.1 Laplace Transforms of First and Second Order Equations 4.2 Fourier Analysis and Fourier Fit Approximations 5. Graphical Analysis of Differential Equations 5.1 Euler's Method 5.2 Flow Plots and Trajectories 5.3 Preditor-Prey Model 5.4 Logistic Harvesting 6. First-Order Differential Equations 6.1 Autonomous Equations 6.2 Non-Autonomous Equations 6.3 Separation of Variables Solving Method 7. Systems of Differential Equations 7.1 Flows and Trajectories 7.2 Conversion Between Higher Order ODEs and Systems 7.3 Relationship to Eigenvalues and Eigenvectors 8. Power Series Solutions of Differential Equations 8.1 Recursion Relations 8.2 Comparing Series Solution to Numerical Solution 8.3 Barriers
Legacy Course Connection
Legacy Distance Calculus Course:
DMAT 317 - Differential Equations
In 2023, Distance Calculus introduced a new catalog of courses. The connection between the old courses and the new courses are given here:
Legacy Course Description: Studies methods of solution of ordinary differential equations with applications in science and engineering. Extensive use is made of the method of Laplace transforms. (3 credits)
| Common Completion Timelines for DMAT 321 - Differential Equations | ||||
| Hours Dedicated | Math Skills | Dedication | Completion Time | Advisory |
|---|---|---|---|---|
| 5-10 hours/week | Weaker | 1-2 hours/day | 14 weeks | Reasonable |
| 7-12 hours/week | Modest | 2-3 hours/day | 10 weeks | Reasonable |
| 10-15 hours/week | Stronger | 3-4 hours/day | 7 weeks | Reasonable |
| 15-20 hours/week | Strong | 5-6 hours/day | 5 weeks | Stretched |
| 20-25 hours/week | Strong | 5-7 hours/day | 4 weeks | Stretched |
| 25-35 hours/week | Strong | 6-8 hours/day | 3 weeks | World's Record |
Time commitments are important for success in an online Differential Equations course for college credit from Distance Calculus. There are no fixed due dates in the Distance Calculus online courses, so it is important that students instead set their schedules for a dedicated amount of time towards the coursework.
It is also very important to consider that going faster through a course is DIRECTLY DEPENDENT upon your math skill level, and your successful engagement of the course. We require that you complete the course in a Mastery Learning format. If you are struggling with the course content, or trying to go too fast where the quality of your submitted work is suffering, then the instructors will force a slow-down of your progress through the course, even if you have fixed deadlines.
High School Students - Differential Equations Course
Many advanced high school students who have completed their AP Calculus AB and BC courses, which are equivalent to Calculus I and Calculus II, respectively, often find themselves in their senior year of high school with no further courses in mathematics to take at their high school.
There are no further AP Calculus courses beyond the AP Calculus BC (Calculus II) course.
An excellent next academic move for these advanced high school mathematics students is to take one or more of the following Distance Calculus courses:
- DMAT 355 - Multivariable Calculus
- DMAT 321 - Differential Equations
- DMAT 335 - Linear Algebra
- DMAT 311 - Probability Theory (Calculus-Based Statistics)
Here is a video discussing some options for these advanced high school students.
Advanced High School Math Plan for Senior Year
After AP Calculus
Differential Equations Online Course Credit: 3 semester university-level credit hours
What are Ordinary Differential Equations?
"Ordinary" means that the functions we are studying are functions of one variable. Usually: y = f(x)
Historically, this course was called "Ordinary Differential Equations", often abbreviated as ODE, and you will see ODE used interchangeably with DE to abbreviate the term "Differential Equations".
What is a non-Ordinary Differential Equation? Those are called Partial Differential Equations, and they involve looking at equations involving partial derivatives of functions of two or more variables, like this: z = f(x,y).
Partial Differential Equations is usually a junior-level mathematics course that all mathematics majors, and many physics and engineering majors, will take to learn about the next steps in the study of differential equations.
The term "ordinary" in Ordinary Differential Equations helps you identify the course level of the Differential Equations course you might be required to take. This Differential Equations online course - DMAT 321 - is the first course on ordinary differential equations.
Colloquially, you may hear other students refer to this course as "Diff-E-Q" or "DiffyQ".
There is also a higher, junior level course on Differential Equations - usually referred to "Advanced Differential Equations", or "Intermediate Differential Equations", which does continue the study of ordinary differential equations, but at a more advanced level, and quite distinct from the partial differential equations courses. Our DMAT 321 - Differential Equations is not considered to be one of these higher junior-level and above courses.
Differential Equations Examples of the Curriculum
Below are some PDF "print outs" of a few of the Mathematica™ notebooks from Differential Equations&Mathematica by Carpenter/Davis/Uhl. Included as well is an example homework notebook completed by a student in the course, demonstrating how the homework notebooks become the "common blackboards" that the students and instructor both write on in their "conversation" about the notebook.
- Sample Curriculum PDF DE.01.B3 - Basics - Steady State Solutions
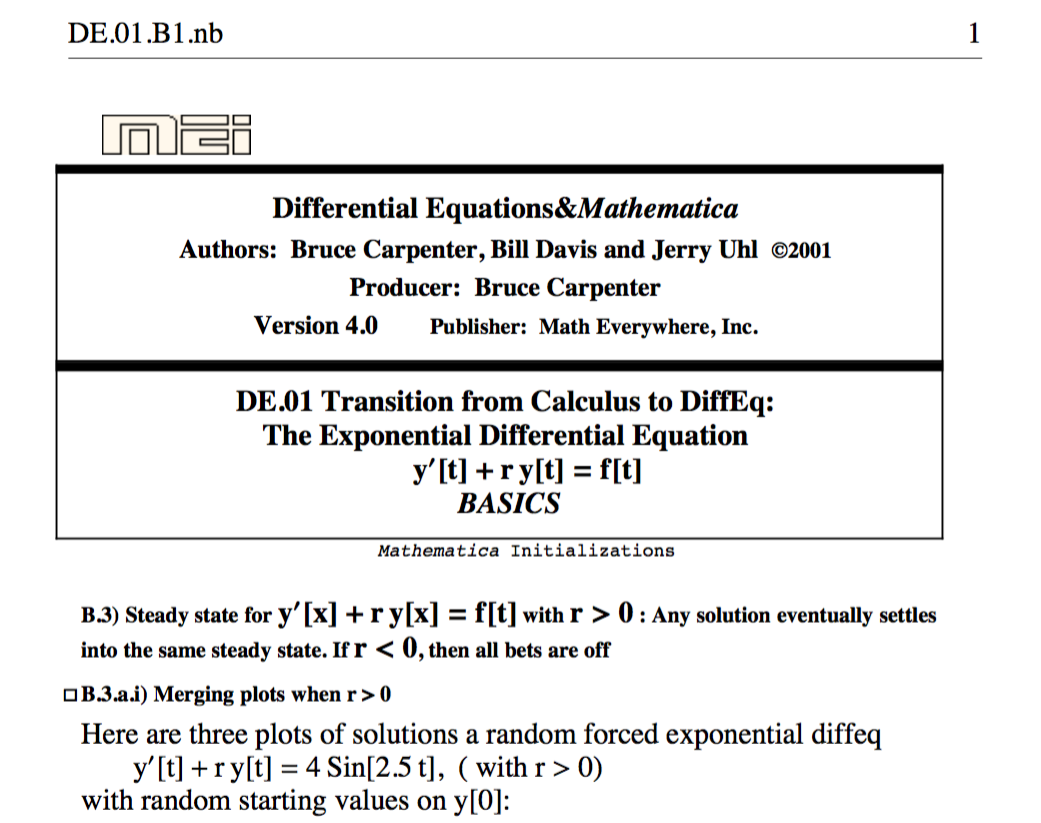
- Screencast Help Video
- Homework Notebook Example PDF DE.02.G1 - Using the Convolution Integral

That Looks Like Programming Code!
Yes, Mathematica™ is a syntax-based computer algebra system - i.e. the instructions to generate the graphs and computations look like a programming language code (which it is).This course is not a course on programming. We do not teach programming, nor do we expect the students to learning programming, or even to know anything about programming. The mathematics is what is important in this course, not the code.
With that tenet in mind, the authors of the Differential Equations&Mathematica courseware have designed the explanation notebooks (Basics & Tutorials) and the homework notebooks (Give It a Try) in such a way as to make it easy to Copy/Paste from the explanations into the homework notebooks, and make minor changes (obvious ones) to produce the desired similar (but different) output. In this way, we are able to stick strictly to the mathematics at hand, and deal with the programming code as minimally as possible.

Differential Equations Online Course Credit: 3 semester university-level credit hours
Distance Calculus - Student Reviews





Date Posted: Apr 30, 2020
Review by: Hannah J.
Courses Completed: Probability Theory
Review: Probability Theory was a great course. Very very thorough. I thought it would never end :). I was very prepared for my coursework in economics. Excellent refereshher of derivatives and integrals - really forced me to remember that stuff from freshman cal.
Transferred Credits to: Boston University





Date Posted: Dec 8, 2020
Review by: Aileen C.
Courses Completed: Differential Equations
Review: This course may be more difficult than your average differential equations course, which better prepares you to use these skills in your degree. The self-learning does make learning some of the concepts challenging, but you get the help you need to understand these concepts.
Transferred Credits to: Johns Hopkins University





Date Posted: Dec 8, 2020
Review by: Aileen C.
Courses Completed: Differential Equations
Review: This course may be more difficult than your average differential equations course, which better prepares you to use these skills in your degree. The self-learning does make learning some of the concepts challenging, but you get the help you need to understand these concepts.
Transferred Credits to: Johns Hopkins University
Frequently Asked Questions
Yes. In some ways Differential Equations is the next course after Calculus II, basically the next course in integration theory, now made more complicated by having more involved equations of derivatives of functions.
The term "Ordinary" simply refers to these functions involving a single variable. The study of differential equations with functions of 2 or more variables is called Partial Differential Equations, because they involve partial derivatives.
Yes. Surprisingly, Differential Equations is usually not required as a prerequisite for Data Science programs, but even though not technically on the requirement list, it is a good idea to take all of the Calculus sequence courses in preparation for a Data Science certificate or degree.
No. Calculus II is very much a prerequisite for Differential Equations, and not a nominal prerequisite. Differential Equations relies very strongly on the mastery of the Calculus II content.
Yes, All Distance Calculus courses are offered through Roger Williams University in Providence, Rhode Island, USA, which is regionally accredited (the highest accreditation) through New England Commission of Higher Education (NECHE).
 Freshman Math Courses
Freshman Math Courses
- Applied Calculus for Business [3 credits] [3CR]
- Applied Calculus for Life Science [3 credits] [3CR]
- Calculus I[4 credits] [4CR]
- Calculus II[4 credits] [4CR]
 Sophomore Math Courses
Sophomore Math Courses
- Multivariable Calculus III [4 credits] [4CR]
- Differential Equations [3 credits] [3CR]
- Linear Algebra [4 credits] [4CR]
- Probability Theory [3 credits] [3CR]
 Honors Math Courses
Honors Math Courses
- Honors Calculus I [5 credits] [5CR]
- Honors Calculus II [5 credits] [5CR]
- Honors Calculus I+II for Data Science [5 credits] [5CR]
- Honors Multivariable Calculus [5 credits] [5CR]
- Honors Differential Equations [4 credits] [4CR]
- Honors Linear Algebra [5 credits] [5CR]
- Honors Linear Algebra for Data Science [5 credits] [5CR]
 Lower Division Math Courses
Lower Division Math Courses
- Precalculus with Trigonometry [4 credits] [4CR]
- Introductory Statistics [4 credits] [4CR]
- Finite Mathematics [3 credits] [3CR]
- Discrete Mathematics [4 credits] [4CR]
 Upper Division Math Courses
Upper Division Math Courses
- Computational Abstract Algebra [4 credits] [4CR]
- Computational Differential Geometry [4 credits] [4CR]

